-
 Raman effect
Raman effect
-
 Rachis
Rachis
-
 Restriction enzymes
Restriction enzymes
-
 SLAC
SLAC
-
 Esker
Esker
-
 Heterozygote
Heterozygote
-
 Solid solution
Solid solution
-
 Modulation
Modulation
-
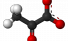 Metabolic crossover
Metabolic crossover
-
 Nutrient
Nutrient
-
 Tholin
Tholin
-
 Celestial meridian
Celestial meridian
-
 Mango
Mango
-
 GMO
GMO
-
 Service tree
Service tree
-
 XOR
XOR
-
 SARS-CoV
SARS-CoV
-
 Podzol
Podzol
-
 Gastrulation
Gastrulation
-
 In vitro fertilisation
In vitro fertilisation
-
 Ventricular fibrillation
Ventricular fibrillation
-
 Plutonic
Plutonic
-
 Trojan horse
Trojan horse
-
 Rydberg atom
Rydberg atom
-
 Plutoid
Plutoid
-
 Scraping
Scraping
-
 ISO 14.001
ISO 14.001
-
 Sessile oak
Sessile oak
-
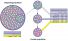 Genetic drift
Genetic drift
-
 Landslide
Landslide
Golden number
The golden number is the value of a certain proportion or ratio between two quantities of the same nature such as two lengths, two angles, two numbers of branches etc.
This number, like "pi", is irrational. Its symbol is "phi" (from Phydias). Its value is given by the solution to the second degree equation x2 - x - 1 = 0 of which the roots are: (1+/- root 5) / 2 = x= 1.61803... and x' = - 0.61803...= - 1 / x .
Any Fibonacci series can be used to find the golden number to at least 2 decimal places from the seventh or eighth number onward. You would have to take the series to infinity to find the exact figures.
In geometry too, the golden number can be found in any regular pentagonal shape and in a 5-pointed star, and in the logarithmic or Archimedean spirals and certain specific patterns.
Latest
Fill out my online form.



