-
 Albuginea
Albuginea
-
 VSC, PVC
VSC, PVC
-
 Accretionary prism
Accretionary prism
-
 Heterolysis
Heterolysis
-
 Endosperm
Endosperm
-
 Dendritic growth
Dendritic growth
-
 Vector processor
Vector processor
-
 HDLC
HDLC
-
 Affinity
Affinity
-
 Magnetopause
Magnetopause
-
 Critical temperature
Critical temperature
-
 Hash function
Hash function
-
 TNM Classification
TNM Classification
-
 Axial skeleton
Axial skeleton
-
 DVD-R
DVD-R
-
 Supercluster
Supercluster
-
 Parenchyma
Parenchyma
-
 Mumps virus
Mumps virus
-
 Billings Method
Billings Method
-
 Vacuum
Vacuum
-
 Potato
Potato
-
 Planet
Planet
-
 Leaving group
Leaving group
-
 Ecological corridor
Ecological corridor
-
 Ephemeral
Ephemeral
-
 Echocardiography
Echocardiography
-
 Antioxidants
Antioxidants
-
 Stereochemical formula
Stereochemical formula
-
 Life expectancy
Life expectancy
-
 Afferent
Afferent
Fractal
A fractal figure, or "fractal ", is to a first approximation a curve, a surface, a volume of irregular or fragmented form created by following deterministic or stochastic rules that imply internal homothety. This term "fractal" is a neologism created by the mathematician Benoît Mandelbrot in 1974 from the Latin root fractus, meaning broken, irregular.
More generally, a fractal designates objects whose structure is invariant by change of scale. In fact there is a precise mathematical theory behind these different objects in which we speak of mathematical structures with non-integer dimensions.
Fractal structures were known before their popularisation at the beginning of the 1980s, thanks to images calculated by computers which by then had become sufficiently powerful. Thus the Peano and von Koch curves were known. Today, the Mandelbrot set and the Julia set are the most well-known.
Fractals in many fields
Approximate Fractal forms are readily observable in nature. The mathematical fractal theory can provide information that is useful in several scientific fields such as:
- in geology, the study of relief, coasts and waterways, rock structures avalanches;
- in animal morphology, structures of invertebrates, birds' feathers;
- in medicine, the structure of lungs, intestines, heart beats;
- in meteorology, clouds, vortices, ice floes, rogue waves, turbulence, the structure of lightning;
- in vulcanology, the prediction of volcanic eruptions, earthquakes;
- in astronomy with the description of structures in the universe, lunar craters, the distribution of galaxies;
- in human science, urban structure, demographic evolution;
- in economics, the prediction of stock exchange crashes.
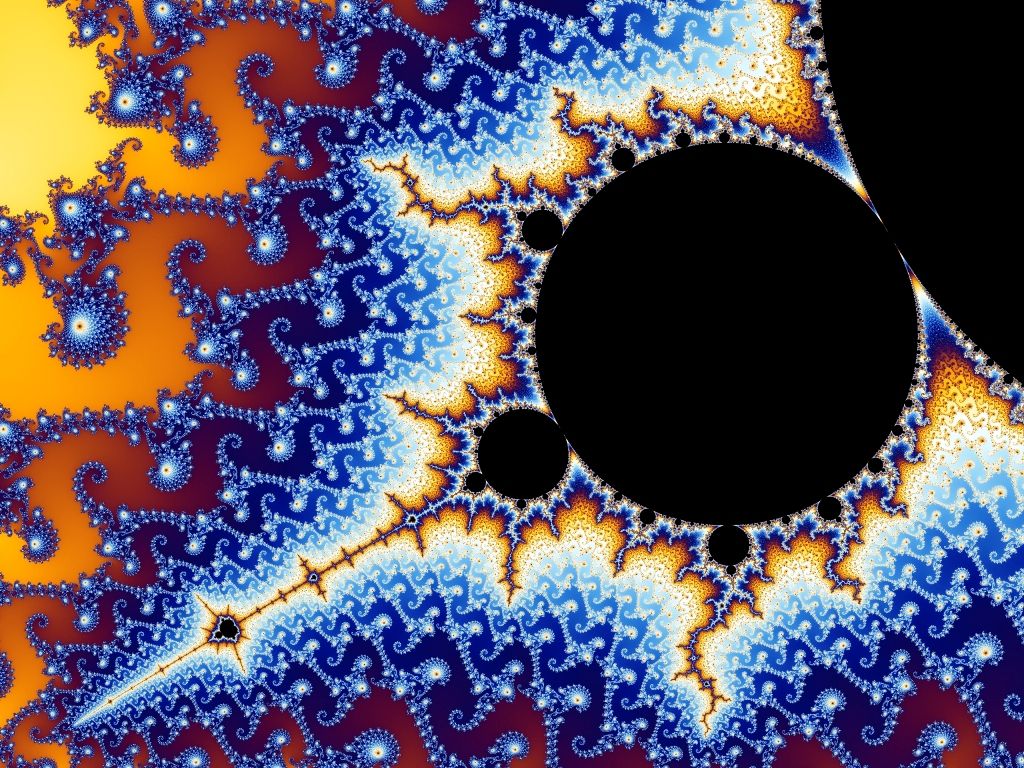 The Mandelbrot set, symbolic of fractals. © wikipedia-commons
The Mandelbrot set, symbolic of fractals. © wikipedia-commons
Latest
Fill out my online form.



